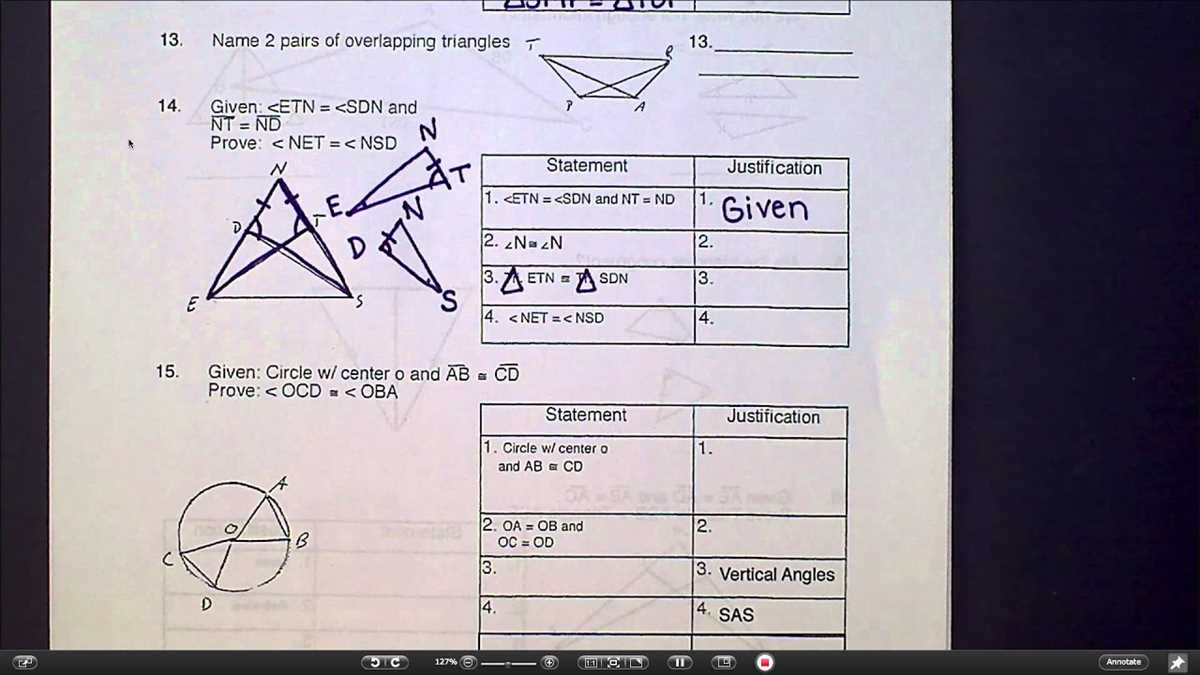
Geometry is the study of shapes, angles, and spatial relationships, and Chapter 7 delves into the intricate concepts of triangles and polygons. In this article, we will provide a comprehensive review of the answers to Chapter 7 to help you solidify your understanding of these fundamental geometric principles.
One of the key topics covered in Chapter 7 is triangle classification. Triangles can be classified based on their angles and sides, and it is crucial to recognize and differentiate between different types of triangles, such as acute, obtuse, right, scalene, isosceles, and equilateral triangles. Our review answers will guide you through the process of identifying the properties and characteristics of each type.
Polygons, another important aspect of Chapter 7, are two-dimensional shapes with straight sides. Understanding the properties and characteristics of polygons is essential for solving geometry problems. This review will provide you with detailed explanations of concepts such as regular and irregular polygons, convex and concave polygons, and the sum of interior angles in polygons.
As you progress through this article, you will find concise answers and explanations to the review questions in Chapter 7. Each answer is carefully crafted to ensure a clear understanding of the principles discussed. Whether you are preparing for an exam or simply seeking to reinforce your knowledge, this review guide will equip you with the necessary tools to excel in geometry.
Geometry Chapter 7 Review Answers
If you’re looking for the answers to the review questions in Chapter 7 of your geometry textbook, you’ve come to the right place. This chapter focuses on advanced topics in geometry, including triangles, circles, and proofs. By reviewing these answers, you can ensure that you have a solid understanding of the material covered in class.
Here are the answers to some of the key review questions in Chapter 7:
- Question 1: What is the Pythagorean Theorem?
- Answer: The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
- Question 2: What is the relationship between the radii of a circle?
- Answer: The radii of a circle are all equal in length, as they all extend from the center of the circle to its perimeter.
- Question 3: How do you prove that two triangles are congruent?
- Answer: Two triangles are congruent if their corresponding sides and angles are equal in length and measure.
By reviewing these answers and practicing additional problems, you can feel confident in your ability to succeed in your geometry class. Remember to always show your work and use proper notation when solving problems. Good luck!
Understanding Quadrilaterals
In geometry, a quadrilateral is a polygon with four sides and four angles. It is a four-sided shape that can have different types and properties based on its angles and side lengths. Understanding the characteristics of quadrilaterals is important in geometry as it helps in identifying and classifying different types of shapes.
Types of Quadrilaterals:
- Rectangle: A rectangle is a quadrilateral with four right angles. It has opposite sides that are equal and parallel. The diagonals of a rectangle are also equal in length and bisect each other.
- Square: A square is a special type of rectangle with all sides of equal length. It has four right angles and its diagonals are equal in length and bisect each other.
- Parallelogram: A parallelogram is a quadrilateral with opposite sides that are parallel. Its opposite angles are also equal. The diagonals of a parallelogram bisect each other.
- Rhombus: A rhombus is a parallelogram with all sides of equal length. It has opposite angles that are equal and its diagonals bisect each other at right angles.
- Trapezoid: A trapezoid is a quadrilateral with one pair of opposite sides that are parallel. Its non-parallel sides can have different lengths.
Understanding the properties and characteristics of different types of quadrilaterals is essential in geometry as it helps in solving problems, proving theorems, and analyzing geometric shapes. It enables us to categorize and classify shapes based on their properties, which makes it easier to study and understand the world of geometry.
Properties of Parallelograms

A parallelogram is a four-sided polygon where opposite sides are parallel. There are several important properties of parallelograms that are worth noting:
- Opposite sides are congruent: In a parallelogram, the lengths of the opposite sides are equal. This means that if one side measures, for example, 5 units, then the opposite side will also measure 5 units.
- Opposite angles are congruent: Another property of parallelograms is that opposite angles are congruent. This means that if one angle measures, for example, 40 degrees, then the angle opposite to it will also measure 40 degrees.
- Consecutive angles are supplementary: The consecutive angles of a parallelogram are supplementary, which means that they add up to 180 degrees. For example, if one angle measures 60 degrees, then the angle next to it will measure 120 degrees.
- Diagonals bisect each other: The diagonals of a parallelogram bisect each other. This means that they divide each other into two equal parts. If the length of a diagonal is 10 units, then each part will measure 5 units.
These properties apply to all parallelograms, regardless of their size or shape. Understanding these properties can help in solving problems involving parallelograms and can provide insights into their geometric properties.
Tests for Parallelograms
Pondering over whether a quadrilateral is a parallelogram or not? There are several tests you can use to determine if a quadrilateral is a parallelogram or not. Here are some of the most common ones:
Opposite sides test: If the opposite sides of a quadrilateral are congruent, then it is a parallelogram. This means that the length of one pair of opposite sides must be equal to the length of the other pair of opposite sides.
Opposite angles test: If the opposite angles of a quadrilateral are congruent, then it is a parallelogram. This means that the measure of one pair of opposite angles must be equal to the measure of the other pair of opposite angles.
- Consecutive angles test: If the consecutive angles of a quadrilateral are supplementary, i.e., they add up to 180 degrees, then it is a parallelogram.
- Diagonals test: If the diagonals of a quadrilateral bisect each other, i.e., they divide each other into two equal parts, then it is a parallelogram.
- Midpoint test: If the midpoints of the sides of a quadrilateral are connected and form a parallelogram, then the original quadrilateral is also a parallelogram.
- Angle bisectors test: If the angle bisectors of the angles of a quadrilateral are concurrent, i.e., they intersect at one point, then it is a parallelogram.
Properties of Trapezoids and Kites
A trapezoid is a quadrilateral with at least one pair of parallel sides. The parallel sides are called bases, and the non-parallel sides are called legs. One important property of trapezoids is that the opposite angles are supplementary, meaning they add up to 180 degrees. Another property is that the diagonals of a trapezoid bisect each other.
Kites, on the other hand, are quadrilaterals with two pairs of congruent adjacent sides. They have one pair of opposite angles that are equal. One key property of kites is that the diagonals are perpendicular to each other. Additionally, the longer diagonal of a kite bisects the shorter diagonal.
In summary, trapezoids and kites have distinct properties that differentiate them from other quadrilaterals. Trapezoids have at least one pair of parallel sides, and their opposite angles are supplementary. The diagonals of a trapezoid bisect each other. Kites have two pairs of congruent adjacent sides and one pair of opposite angles that are equal. The diagonals of a kite are perpendicular to each other, and the longer diagonal bisects the shorter diagonal.
The Pythagorean Theorem and its Converse
The Pythagorean Theorem is a fundamental concept in geometry that states that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. This theorem is named after the ancient Greek mathematician Pythagoras, who is credited with its discovery.
The Pythagorean Theorem can be represented as the equation a^2 + b^2 = c^2, where a and b are the lengths of the legs of the right triangle, and c is the length of the hypotenuse. This theorem is incredibly useful in solving problems involving right triangles, as it allows us to determine the length of a missing side or the measure of an angle.
- For example, if we have a right triangle with one leg measuring 3 units and the hypotenuse measuring 5 units, we can use the Pythagorean Theorem to find the length of the other leg. We know that 3^2 + b^2 = 5^2, so 9 + b^2 = 25. Solving for b, we find that b = 4 units.
- The converse of the Pythagorean Theorem states that if the square of one side of a triangle is equal to the sum of the squares of the other two sides, then the triangle is a right triangle. This allows us to determine if a given triangle is a right triangle based on its side lengths.
Knowing the Pythagorean Theorem and its converse is essential for solving problems involving right triangles and understanding the relationships between their sides. It is a powerful tool that has applications in various fields, including architecture, engineering, and physics.
Special Right Triangles
In geometry, special right triangles are a category of right triangles that have specific properties and angles that make them easier to work with and solve. These triangles have angles that are always the same and ratios between their sides that are consistent.
There are two main types of special right triangles: the 45-45-90 triangle and the 30-60-90 triangle. The 45-45-90 triangle, also known as an isosceles right triangle, has two equal angles of 45 degrees and one right angle of 90 degrees. The sides in this triangle have specific ratios: the length of the hypotenuse is always equal to the length of one of the legs times the square root of 2, and the length of each leg is equal to each other.
The 30-60-90 triangle, also known as a right triangle with special ratios, has angles of 30 degrees, 60 degrees, and 90 degrees. The ratios between its sides are also specific: the length of the hypotenuse is always twice the length of the shorter leg, and the length of the longer leg is equal to the length of the shorter leg times the square root of 3.
Special right triangles are useful in geometry because their specific ratios and angles allow for easier calculation of side lengths and angle measurements. They are often used in trigonometry and more advanced geometric calculations. Understanding the properties of these triangles can be helpful in solving problems involving right triangles and finding missing side lengths and angle measures.
The Distance and Midpoint Formulas
The distance formula is a mathematical formula used to find the distance between two points in a coordinate plane. It is derived from the Pythagorean theorem and applies to any two points (x1, y1) and (x2, y2). The formula is:
d = √[(x2 – x1)^2 + (y2 – y1)^2]
Where d represents the distance between the two points. This formula can be used to find the distance between any two points in a coordinate plane.
The midpoint formula, on the other hand, is used to find the coordinates of the midpoint of a line segment. The coordinates of the midpoint can be found by taking the average of the x-coordinates and the average of the y-coordinates of the endpoints. The formula is:
(x, y) = ((x1 + x2) / 2, (y1 + y2) / 2)
Where (x, y) represents the coordinates of the midpoint and (x1, y1) and (x2, y2) represent the coordinates of the endpoints of the line segment. This formula is useful in determining the center point of a line segment.
Circles and their Constructions
In geometry, a circle is defined as a set of all points in a plane that are equidistant from a fixed center point. Circles are one of the most fundamental shapes studied in mathematics, and their properties and constructions are extensively explored.
One of the key characteristics of a circle is its radius, which is the distance from the center to any point on the circle. The radius is constant for all points on the circle. Another important property is the diameter, which is the distance across the circle passing through the center. The diameter is always twice the length of the radius.
Circle constructions involve various techniques to create circles and their related elements. These constructions are performed using only a compass and a straightedge, without relying on measurements or specific numerical values. Construction techniques include creating a circle with a given radius, constructing tangents to a circle, and inscribing polygons within circles.
To construct a circle with a given radius, one can use a compass to draw an arc with the desired length from a specific point. Another construction technique involves constructing tangents to a circle. Tangents are lines that intersect a circle at exactly one point, and they can be constructed using a compass and straightedge.
In addition to circles themselves, constructions can also be used to inscribe or circumscribe polygons within circles. For example, one can use a compass and straightedge to construct a triangle or a regular hexagon inside a given circle. These constructions allow for the exploration of relationships between circles and polygons, as well as the inscribed and circumscribed angles and lengths.